0.3 Factoring Expressions
Now that we have reviewed the basics of polynomial arithmetic it’s time to review the basic techniques of factoring polynomial expressions. Our goal is to apply these techniques to help us solve certain specialized classes of non-linear equations. Given that `factoring’ literally means to resolve a product into its factors, it is, in the purest sense, `undoing’ multiplication. If this sounds like division to you then you’ve been paying attention. Let’s start with a numerical example.
Suppose we are asked to factor ![]() . We could write
. We could write ![]() , and while this is technically a factorization of
, and while this is technically a factorization of ![]() , it’s probably not an answer the poser of the question would accept. Usually, when we’re asked to factor a natural number, we are being asked to resolve it into to a product of so-called `prime’ numbers.[1] Recall that prime numbers are defined as natural numbers whose only (natural number) factors are themselves and
, it’s probably not an answer the poser of the question would accept. Usually, when we’re asked to factor a natural number, we are being asked to resolve it into to a product of so-called `prime’ numbers.[1] Recall that prime numbers are defined as natural numbers whose only (natural number) factors are themselves and ![]() . They are, in essence, the `building blocks’ of natural numbers as far as multiplication is concerned. Said differently, we can build – via multiplication – any natural number given enough primes.
. They are, in essence, the `building blocks’ of natural numbers as far as multiplication is concerned. Said differently, we can build – via multiplication – any natural number given enough primes.
So how do we find the prime factors of ![]() ? We start by dividing each of the primes:
? We start by dividing each of the primes: ![]() ,
, ![]() ,
, ![]() ,
, ![]() , etc., into
, etc., into ![]() until we get a remainder of
until we get a remainder of ![]() . Eventually, we determine that
. Eventually, we determine that ![]() with a remainder of
with a remainder of ![]() , which means
, which means ![]() . So factoring and division are indeed closely related – factors of a number are precisely the divisors of that number which produce a zero remainder.[2] We continue our efforts to see if
. So factoring and division are indeed closely related – factors of a number are precisely the divisors of that number which produce a zero remainder.[2] We continue our efforts to see if ![]() can be factored down further, and we find that
can be factored down further, and we find that ![]() . Hence,
. Hence, ![]() can be `completely factored’ as
can be `completely factored’ as ![]() . (This factorization is called the prime factorization of
. (This factorization is called the prime factorization of ![]() .)
.)
In factoring natural numbers, our building blocks are prime numbers, so to be completely factored means that every number used in the factorization of a given number is prime. One of the challenges when it comes to factoring polynomial expressions is to explain what it means to be `completely factored’. In this section, our `building blocks’ for factoring polynomials are `irreducible’ polynomials as defined below.
Definition 0.4
A polynomial is said to be irreducible if it cannot be written as the product of polynomials of lower degree.
While Definition 0.4 seems straightforward enough, sometimes a greater level of specificity is required. For example, ![]() . While
. While ![]() and
and ![]() are perfectly fine polynomials, factoring which requires irrational numbers is usually saved for a more advanced treatment of factoring. For now, we will restrict ourselves to factoring using rational coefficients. So, while the polynomial
are perfectly fine polynomials, factoring which requires irrational numbers is usually saved for a more advanced treatment of factoring. For now, we will restrict ourselves to factoring using rational coefficients. So, while the polynomial ![]() can be factored using irrational numbers, it is called irreducible over the rationals, because there are no polynomials with rational coefficients of smaller degree which can be used to factor it.[3]
can be factored using irrational numbers, it is called irreducible over the rationals, because there are no polynomials with rational coefficients of smaller degree which can be used to factor it.[3]
Due to the fact that polynomials involve terms, the first step in any factoring strategy involves pulling out factors which are common to all of the terms. For example, in the polynomial ![]() , each coefficient is a multiple of
, each coefficient is a multiple of ![]() so we can begin the factorization as
so we can begin the factorization as ![]() . The remaining coefficients:
. The remaining coefficients: ![]() ,
, ![]() and
and ![]() , have no common factors so
, have no common factors so ![]() was the greatest common factor. What about the variables? Each term contains an
was the greatest common factor. What about the variables? Each term contains an ![]() , so we can factor an
, so we can factor an ![]() from each term. When we do this, we are effectively dividing each term by
from each term. When we do this, we are effectively dividing each term by ![]() which means the exponent on
which means the exponent on ![]() in each term is reduced by
in each term is reduced by ![]() :
: ![]() . Next, we see that each term has a factor of
. Next, we see that each term has a factor of ![]() in it. In fact, each term has at least two factors of
in it. In fact, each term has at least two factors of ![]() in it, as the lowest exponent on
in it, as the lowest exponent on ![]() in each term is
in each term is ![]() . This means that we can factor
. This means that we can factor ![]() from each term. Again, factoring out
from each term. Again, factoring out ![]() from each term is tantamount to dividing each term by
from each term is tantamount to dividing each term by ![]() so the exponent on
so the exponent on ![]() in each term is reduced by two:
in each term is reduced by two: ![]() . Just like we checked our division by multiplication in the previous section, we can check our factoring here by multiplication, too.
. Just like we checked our division by multiplication in the previous section, we can check our factoring here by multiplication, too.
![]()
We summarize how to determine the Greatest Common Factor (G.C.F.) of a polynomial expression below.
Determining the G.C.F. of a Polynomial Expression
- If the coefficients are integers, identify the G.C.F. of the coefficients.
Note 1: If all of the coefficients are negative, consider the negative as part of the G.C.F..
Note 2: If the coefficients involve fractions, get a common denominator, combine numerators, reduce to lowest terms and apply this step to the polynomial in the numerator. - If a variable is common to all of the terms, the G.C.F. contains that variable to the smallest exponent which appears among the terms.
For example, to factor ![]() , we would first get a common denominator and factor as:
, we would first get a common denominator and factor as:
![Rendered by QuickLaTeX.com \[ \begin{array}{rcl} -\frac{3}{5}z^3 - 6z^2 &=& \frac{-3z^3 - 30z^2}{5} \\[5pt] &=& \frac{-3z^2(z + 10)}{5} \\[5pt] &=& -\frac{3z^2(z + 10)}{5} \\[5pt] &=& -\frac{3}{5}z^{2}(z + 10) \end{array}\]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-f78edcf7733b5fdd6d5f1135240ea905_l3.png)
We now list some common factoring formulas, each of which can be verified by multiplying out the right side of the equation. While they all should look familiar – this is a review section after all – some should look more familiar than others because they are `special product’ formulas.
Common Factoring Formulas
- Perfect Square Trinomials:
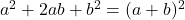 and
and 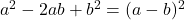
- Difference of Two Squares:

Note: In general, the sum of squares,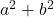 is irreducible over the rationals.
is irreducible over the rationals. - Sum of Two Cubes:
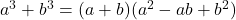
Note: In general, is irreducible over the rationals.
is irreducible over the rationals. - Difference of Two Cubes:
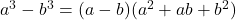
Note: In general, is irreducible over the rationals.
is irreducible over the rationals.
The example on the next page gives us practice with these formulas.
Example 0.3.1
Example 0.3.1.1
Factor the following polynomials completely over the rationals. That is, write each polynomial as a product polynomials of lowest degree which are irreducible over the rationals.
![]()
Solution:
Factor ![]() .
.
Our first step is to factor out the G.C.F. which in this case is ![]() . To match what is left with one of the special forms, we rewrite
. To match what is left with one of the special forms, we rewrite ![]() and
and ![]() . We see that we have a perfect square trinomial, because the `middle’ term is
. We see that we have a perfect square trinomial, because the `middle’ term is ![]() .
.
![Rendered by QuickLaTeX.com \[ \begin{array}{rclr} 18x^2 - 48x + 32 & = & 2(9x^2 - 24x + 16) & \text{Factor out G.C.F.}\\ & = & 2((3x)^2 - 2(4)(3x) + (4)^2) & \\ & = & 2(3x-4)^2 & \text{Perfect Square Trinomial: $a = 3x$, $b=4$} \\ \end{array}\]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-154849886bbc61b695c69870a17a0c1b_l3.png)
Our final answer is ![]() .
.
To check our work, we multiply out ![]() to show that it equals
to show that it equals ![]() .
.
Example 0.3.1.2
Factor the following polynomials completely over the rationals. That is, write each polynomial as a product polynomials of lowest degree which are irreducible over the rationals.
![]()
Solution:
Factor ![]() .
.
For ![]() , we note that the G.C.F. of the terms is just
, we note that the G.C.F. of the terms is just ![]() , so there is nothing (of substance) to factor out of both terms.
, so there is nothing (of substance) to factor out of both terms.
Due to the fact that ![]() is the difference of two terms, one of which is a square, we look to the Difference of Squares Formula for inspiration. Seeing
is the difference of two terms, one of which is a square, we look to the Difference of Squares Formula for inspiration. Seeing ![]() and
and ![]() , we get
, we get
![]()
As before, we can check our final answer by multiplying out ![]() to show that it equals
to show that it equals ![]() .
.
Example 0.3.1.3
Factor the following polynomials completely over the rationals. That is, write each polynomial as a product polynomials of lowest degree which are irreducible over the rationals.
![]()
Solution:
Factor ![]() .
.
The G.C.F. of the terms in ![]() is
is ![]() , so we factor that out first.
, so we factor that out first.
![]()
We identify what remains as a perfect square trinomial:
![]()
Our final answer is ![]() , which the reader is invited to check.
, which the reader is invited to check.
Example 0.3.1.4
Factor the following polynomials completely over the rationals. That is, write each polynomial as a product polynomials of lowest degree which are irreducible over the rationals.
![]()
Solution:
Factor ![]() .
.
For ![]() , we identify the G.C.F. as
, we identify the G.C.F. as ![]() and once we factor it out a difference of cubes is revealed:
and once we factor it out a difference of cubes is revealed:
![]()
Our final answer is ![]() .
.
The reader is strongly encouraged to multiply this out to see that it reduces to ![]() .
.
Example 0.3.1.5
Factor the following polynomials completely over the rationals. That is, write each polynomial as a product polynomials of lowest degree which are irreducible over the rationals.
![]()
Solution:
Factor ![]() .
.
The G.C.F. of the terms in ![]() is just
is just ![]() so there is nothing of substance to factor out from both terms.
so there is nothing of substance to factor out from both terms.
With just a difference of two terms, we are limited to fitting this polynomial into either the Difference of Two Squares or Difference of Two Cubes formula. With the variable here being ![]() , and
, and ![]() is a multiple of
is a multiple of ![]() , we can think of
, we can think of ![]() . This means that we can write
. This means that we can write ![]() which is a perfect square. (As
which is a perfect square. (As ![]() is not a multiple of
is not a multiple of ![]() , we cannot write
, we cannot write ![]() as a perfect cube of a polynomial.) Identifying
as a perfect cube of a polynomial.) Identifying ![]() and
and ![]() , we apply the Difference of Squares Formula to get:
, we apply the Difference of Squares Formula to get:
![]()
At this point, we have an opportunity to proceed further in the first quantity. Identifying ![]() and
and ![]() , we see that we have another difference of squares in the first quantity, which we can reduce. (The sum of two squares in the second quantity cannot be factored over the rationals.)
, we see that we have another difference of squares in the first quantity, which we can reduce. (The sum of two squares in the second quantity cannot be factored over the rationals.)
![Rendered by QuickLaTeX.com \[ \begin{array}{rclr} 81 - 16t^4 & = & (9-4t^2)(9+4t^2) & \\ & = & (3^2 - (2t)^2) (9 + 4t^2) & \\ & = & (3 - 2t)(3+2t)(9 + 4t^2) & \text{Difference of Squares, $a = 3$, $b = 2t$} \\ \end{array} \]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-55c0b3d3e2ffae91815529bf5a3d3bb3_l3.png)
As always, the reader is encouraged to multiply out ![]() to check the result.
to check the result.
Example 0.3.1.6
Factor the following polynomials completely over the rationals. That is, write each polynomial as a product polynomials of lowest degree which are irreducible over the rationals.
![]()
Solution:
Factor ![]() .
.
With a G.C.F. of ![]() and just two terms,
and just two terms, ![]() is a candidate for both the Difference of Squares and the Difference of Cubes formulas. Notice that we can identify
is a candidate for both the Difference of Squares and the Difference of Cubes formulas. Notice that we can identify ![]() and
and ![]() (both perfect squares), but also
(both perfect squares), but also ![]() and
and ![]() (both perfect cubes). If we follow the Difference of Squares approach, we get:
(both perfect cubes). If we follow the Difference of Squares approach, we get:
![]()
At this point, we have an opportunity to use both the Difference and Sum of Cubes formulas:
![Rendered by QuickLaTeX.com \[ \begin{array}{rclr} x^6 - 64 & = & (x^3 - 2^3)(x^3 + 2^3) & \\ & = & (x-2)(x^2+2x+2^2)(x+2)(x^2 - 2x + 2^2) & \text{Sum / Diff. of Cubes, $a = x$, $b = 2$} \\ & = & (x-2)(x+2)(x^2-2x+4)(x^2+2x+4) & \text{Rearrange factors} \\ \end{array} \]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-56d8ef8b1668b819d0a1dbf023a99a8b_l3.png)
From this approach, our final answer is ![]() .
.
Following the Difference of Cubes Formula approach, we get:
![Rendered by QuickLaTeX.com \[ \begin{array}{rclr} x^6 - 64 & = & (x^2)^3 - 4^3 & \\ & = & (x^2 - 4)((x^2)^2 + 4x^2 + 4^2) & \text{Difference of Cubes, $a = x^2$, $b = 4$} \\ & = & (x^2 - 4)(x^4 + 4x^2 + 16) & \\ \end{array} \]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-45f780ea6322c9f210c21f183c898f59_l3.png)
At this point, we recognize ![]() as a difference of two squares:
as a difference of two squares:
![]()
Unfortunately, the remaining factor ![]() is not a perfect square trinomial – the middle term would have to be
is not a perfect square trinomial – the middle term would have to be ![]() for this to work – so our final answer using this approach is
for this to work – so our final answer using this approach is ![]() .
.
This isn’t as factored as our result from the Difference of Squares approach which was ![]() . While it is true that
. While it is true that ![]()
![]() , there is no `intuitive’ way to motivate this factorization at this point.[4]
, there is no `intuitive’ way to motivate this factorization at this point.[4]
The moral of the story? When given the option between using the Difference of Squares and Difference of Cubes, start with the Difference of Squares.
Our final answer to this problem is ![]() .
.
The reader is strongly encouraged to show that this reduces down to ![]() after performing all of the multiplication.
after performing all of the multiplication.
The formulas above, while useful, can only take us so far. Thus, we need to review some additional factoring strategies which should be good friends from back in the day!
Additional Factoring Formulas
- `un-F.O.I.L.ing’: Given a trinomial
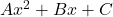 , try to reverse the F.O.I.L. process.
, try to reverse the F.O.I.L. process.
That is, find ,
,  ,
,  and
and 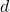 such that
such that 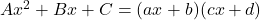 .
.
Note: This means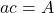 ,
, 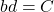 and
and 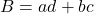 .
. - Factor by Grouping: If the expression contains four terms with no common factors among the four terms, try `factor by grouping’:
![Rendered by QuickLaTeX.com \[ac + bc + ad + bd = (a +b)c + (a+b)d = (a+b)(c+d)\]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-98461ac5329114e5a9b63b09be433b70_l3.png)
The techniques of `un-F.O.I.L.ing’ and `factoring by grouping’ are difficult to describe in general but should make sense to you with enough practice. Be forewarned – like all `Rules of Thumb’, these strategies work just often enough to be useful, but you can be sure there are exceptions which will defy any advice given here and will require some `inspiration’ to solve. Even though Chapter 2 will give us more powerful factoring methods, we’ll find that, in the end, there is no single algorithm for factoring which works for every polynomial. In other words, there will be times when you just have to try something and see what happens.
Example 0.3.2
Example 0.3.2.1
Factor the following polynomials completely over the integers. This means that all of the coefficients in the factors will be integers. While it was decided to avoid fractions in this set of examples, don’t get complacent, though, because fractions will return with a vengeance soon enough.
![]()
Solution:
Factor ![]() .
.
The G.C.F. of the terms ![]() is
is ![]() and
and ![]() isn’t a perfect square trinomial (Think about why not.) so we try to reverse the F.O.I.L. process and look for integers
isn’t a perfect square trinomial (Think about why not.) so we try to reverse the F.O.I.L. process and look for integers ![]() ,
, ![]() ,
, ![]() and
and ![]() such that
such that ![]() .
.
To get started, we note that ![]() . Because
. Because ![]() and
and ![]() are meant to be integers, that leaves us with either
are meant to be integers, that leaves us with either ![]() and
and ![]() both being
both being ![]() , or
, or ![]() and
and ![]() both being
both being ![]() . We’ll go with
. We’ll go with ![]() , as we can factor the negatives into our choices for
, as we can factor the negatives into our choices for ![]() and
and ![]() . This yields
. This yields ![]() .
.
Next, we use the fact that ![]() . The product is negative so we know that one of
. The product is negative so we know that one of ![]() or
or ![]() is positive and the other is negative. Given
is positive and the other is negative. Given ![]() and
and ![]() are integers, one of
are integers, one of ![]() or
or ![]() is
is ![]() and the other is
and the other is ![]() OR one of
OR one of ![]() or
or ![]() is
is ![]() and the other is
and the other is ![]() .
.
After some guessing and checking, we find that ![]() .
.
Example 0.3.2.2
Factor the following polynomials completely over the integers. This means that all of the coefficients in the factors will be integers. While it was decided to avoid fractions in this set of examples, don’t get complacent, though, because fractions will return with a vengeance soon enough.
![]()
Solution:
Factor ![]() .
.
As with the previous example, we check the G.C.F. of the terms in ![]() , determine it to be
, determine it to be ![]() and see that the polynomial doesn’t fit the pattern for a perfect square trinomial.
and see that the polynomial doesn’t fit the pattern for a perfect square trinomial.
We now try to find integers ![]() ,
, ![]() ,
, ![]() and
and ![]() such that
such that ![]() . As
. As ![]() , we have that one of
, we have that one of ![]() or
or ![]() is
is ![]() , and the other is
, and the other is ![]() . (Once again, we ignore the negative options.) At this stage, there is nothing really distinguishing
. (Once again, we ignore the negative options.) At this stage, there is nothing really distinguishing ![]() from
from ![]() so we choose
so we choose ![]() and
and ![]() .
.
Now we look for ![]() and
and ![]() such that
such that ![]() . We know
. We know ![]() so one of
so one of ![]() or
or ![]() is
is ![]() and the other
and the other ![]() . Given that
. Given that ![]() is positive,
is positive, ![]() and
and ![]() must have the same sign. The negative middle term
must have the same sign. The negative middle term ![]() guides us to guess
guides us to guess ![]() and
and ![]() so that we get
so that we get ![]() . We verify our answer by multiplying.[5]
. We verify our answer by multiplying.[5]
Example 0.3.2.3
Factor the following polynomials completely over the integers. This means that all of the coefficients in the factors will be integers. While it was decided to avoid fractions in this set of examples, don’t get complacent, though, because fractions will return with a vengeance soon enough.
![]()
Solution:
Factor ![]() .
.
Once again, we check for a nontrivial G.C.F. and determine if ![]() fits the pattern of a perfect square.
fits the pattern of a perfect square.
Twice disappointed, we rewrite ![]() for notational convenience. We now look for integers
for notational convenience. We now look for integers ![]() ,
, ![]() ,
, ![]() and
and ![]() such that
such that ![]() . Due to the fact that
. Due to the fact that ![]() , we know that one of
, we know that one of ![]() or
or ![]() is
is ![]() and the other
and the other ![]() OR one of them is
OR one of them is ![]() and the other is
and the other is ![]() OR one of them is
OR one of them is ![]() while the other is
while the other is ![]() . As their product is
. As their product is ![]() , however, we know one of them is positive, while the other is negative.
, however, we know one of them is positive, while the other is negative.
To make matters worse, the constant term ![]() has its fair share of factors, too. Our answers for
has its fair share of factors, too. Our answers for ![]() and
and ![]() lie among the pairs
lie among the pairs ![]() and
and ![]() ,
, ![]() and
and ![]() ,
, ![]() and
and ![]() , or
, or ![]() .
.
Because we know one of ![]() or
or ![]() will be negative, we can simplify our choices for
will be negative, we can simplify our choices for ![]() and
and ![]() and just look at the positive possibilities. After some guessing and checking,[6] we determine
and just look at the positive possibilities. After some guessing and checking,[6] we determine ![]() .
.
Example 0.3.2.4
Factor the following polynomials completely over the integers. This means that all of the coefficients in the factors will be integers. While it was decided to avoid fractions in this set of examples, don’t get complacent, though, because fractions will return with a vengeance soon enough.
![]()
Solution:
Factor ![]() .
.
Given the G.C.F. of the terms in ![]() is
is ![]() , we begin the problem by factoring it out first:
, we begin the problem by factoring it out first:
![]()
We now focus our attention on ![]() . We can take
. We can take ![]() and
and ![]() to both be
to both be ![]() which yields
which yields ![]() .
.
Our choices for ![]() and
and ![]() are among the factor pairs of
are among the factor pairs of ![]() :
: ![]() and
and ![]() or
or ![]() and
and ![]() , where one of
, where one of ![]() or
or ![]() is positive and the other is negative. We find
is positive and the other is negative. We find ![]() .
.
Our final answer is ![]() .
.
Example 0.3.2.5
Factor the following polynomials completely over the integers. This means that all of the coefficients in the factors will be integers. While it was decided to avoid fractions in this set of examples, don’t get complacent, though, because fractions will return with a vengeance soon enough.
![]()
Solution:
Factor ![]() .
.
With ![]() being four terms, we are pretty much resigned to factoring by grouping. The strategy here is to factor out the G.C.F. from two pairs of terms, and see if this reveals a common factor.
being four terms, we are pretty much resigned to factoring by grouping. The strategy here is to factor out the G.C.F. from two pairs of terms, and see if this reveals a common factor.
If we group the first two terms, we can factor out a ![]() to get
to get ![]() .
.
We now try to factor something out of the last two terms that will leave us with a factor of ![]() . Sure enough, we can factor out a
. Sure enough, we can factor out a ![]() from both:
from both: ![]() . Hence, we get
. Hence, we get
![]()
Now the question becomes can we factor ![]() over the integers? This would require integers
over the integers? This would require integers ![]() and
and ![]() such that
such that ![]() . As a result of
. As a result of ![]() and
and ![]() , we aren’t left with many options – in fact, we really have only four choices:
, we aren’t left with many options – in fact, we really have only four choices: ![]() ,
, ![]() ,
, ![]() and
and ![]() . None of these produce
. None of these produce ![]() – which means it’s irreducible over the integers.
– which means it’s irreducible over the integers.
Thus our final answer is ![]() .
.
Example 0.3.2.6
Factor the following polynomials completely over the integers. This means that all of the coefficients in the factors will be integers. While it was decided to avoid fractions in this set of examples, don’t get complacent, though, because fractions will return with a vengeance soon enough.
![]()
Solution:
Factor ![]() .
.
Our last example, ![]() , is our old friend from Example 0.3.1. As noted there, it is not a perfect square trinomial, so we could try to reverse the F.O.I.L. process.
, is our old friend from Example 0.3.1. As noted there, it is not a perfect square trinomial, so we could try to reverse the F.O.I.L. process.
This is complicated by the fact that our highest degree term is ![]() , so we would have to look at factorizations of the form
, so we would have to look at factorizations of the form ![]() as well as
as well as ![]() . We leave it to the reader to show that neither of those work.
. We leave it to the reader to show that neither of those work.
This is an example of where `trying something’ pays off. Even though we’ve stated that it is not a perfect square trinomial, it’s pretty close. Identifying ![]() and
and ![]() , we’d have
, we’d have ![]() , but instead of
, but instead of ![]() as our middle term, we only have
as our middle term, we only have ![]() . We could add in the extra
. We could add in the extra ![]() we need, but to keep the balance, we’d have to subtract it off. Doing so produces an unexpected opportunity:
we need, but to keep the balance, we’d have to subtract it off. Doing so produces an unexpected opportunity:
![Rendered by QuickLaTeX.com \[ \begin{array}{rclr} x^4 + 4x^2 + 16 & = & x^4 + 4x^2 + 16 + (4x^2 - 4x^2) & \text{Adding and subtracting the same term} \\ & = & x^4 + 8x^2 + 16 - 4x^2 & \text{Rearranging terms} \\ & = & (x^2 + 4)^2 - (2x)^2 & \text{Factoring perfect square trinomial} \\ & = & [(x^2 +4) - 2x][ (x^2 + 4) + 2x] & \text{Difference of Squares: $a= (x^2 + 4)$, $b = 2x$}\\ & = & (x^2 - 2x + 4)(x^2 + 2x + 4) & \text{Rearraging terms} \\ \end{array}\]](https://pressbooks.library.tamu.edu/app/uploads/quicklatex/quicklatex.com-b36ebbec7d9743d691187028124595e1_l3.png)
We leave it to the reader to check that neither ![]() nor
nor ![]() factor over the integers, so we are done.
factor over the integers, so we are done.
0.3.1 Section Exercises
In Exercises 1 – 30, factor completely over the integers. Check your answer by multiplication.
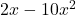
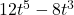
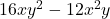
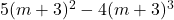
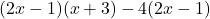
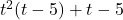
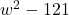
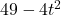
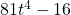
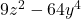
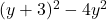

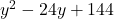
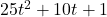
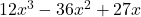
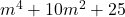
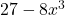
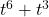
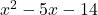
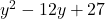
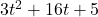
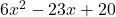
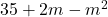
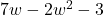
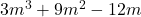

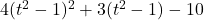
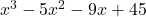
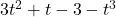
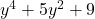 , Note:
, Note: 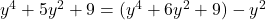
- As mentioned in Section 0.1, this is possible, in only one way, thanks to the Fundamental Theorem of Arithmetic. ↵
- We'll refer back to this when we get to Section 2.3. ↵
- If this isn't immediately obvious, don't worry - in some sense, it shouldn't be. We'll talk more about this later. ↵
- Of course, this begs the question, ``How do we know
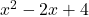 and
and 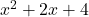 are irreducible?'' (We were told so on previously, but no reason was given.) Stay tuned! We'll get back to this in due course. ↵
are irreducible?'' (We were told so on previously, but no reason was given.) Stay tuned! We'll get back to this in due course. ↵ - That's the `checking' part of 'guessing and checking'. ↵
- Some of these guesses can be more `educated' than others. Due to the fact that the middle term is relatively `small,' we don't expect the `extreme' factors of
 and
and  to appear, for instance. ↵
to appear, for instance. ↵
Natural numbers whose only (natural number) factors are themselves and $1$.
It cannot be written as the product of polynomials of lower degree.

